HomeGalleriesArticlesReferencesForumLinksAbout

Latest galleries :

Flat Earth Nonsense
The so-called 'flat Earth doctrine' debunked in a couple of easy, verifiable steps.
Added on 2018-03-28

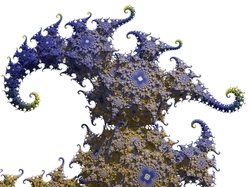3D Kleinian escape time
Images made with an escape time algorithm. All images were created in Ultrafractal.
Added on 2017-09-20

Moebius strip and cross-cap
Visual proof that a Moebius strip is homeomorphic to a cross-cap with a disc removed.
Added on 2016-03-01

Dodecahedral tesselation of the hypersphere
A dissection of the 120 cell in twelve rings of 10 dodecahedra. Two sets of six rings form 2 solid interlocked tori. The film starts by showing the 600 cell, the dual of the 120 cell.
Added on 2016-02-21

Connected sums of real projective plane and torus or Klein bottle.
Visual proof that the connected sum of a real projective plane and a torus , and the connected sum of a real projective plane and a Klein bottle are homeomorphic.
Added on 2016-02-20

View All galleries by date

Galleries by category :

	Kleinian groups Where we enter the wonderful world first envisaged by Felix Klein. These pages where inspired by "Indra's Pearls" by David Mumford, Caroline Series and David Wright.
	3D inversive geometry Where we we find ways of filling spheres with spheres and do other strange things with this simplest of all 3D objects...
	3D Fractals Different species of fractals in 3D...
	Circle Packings Where we discover the art of assembling kissing circles, and find out what Doyle spirals are.
	The M.C.Escher flavoured pages Where we find impossible geometry, tesselations and even the Droste effect.
	Advanced geometry Where we find Penrose, Voronoi and hyperbolic tilings..and where we have some fun with circles and spheres.We also encounter math surfaces, plane-filling curves, knot dynamics and take a mathematical look at the shape of Planet Earth.
	Simple geometry Things like Celtic knots, spirals and 'Looms".
	Fractals Fractal images, including general 3D fractals and the Mandelbulb.
	Movies Movie collection
	Biomorphic fractals Biomorphic: resembling or suggesting the forms of living organisms
	Topology movies Topics in topology